Calculate surface areas and volumes of spheres, pyramids, cones and compound solids (2)
This
is the second of two weekly sets of tasks looking at surface area and
volume of various kinds of solids. Here we focus on the pyramid, though we start with a 'wedge', and the tasks are mostly quantitative.
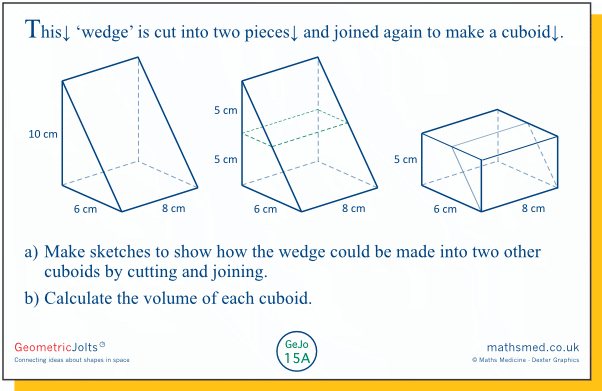
MONDAY: Here we transform a wedge-shape into a cuboid in various ways.
In the given example, the wedge has been transformed into a cuboid with the same base but half the height of the original wedge. We can also transform it into a cuboid with half the base of the original wedge but the same height, as in the examples below. Of course, we don't need to transform the wedge to find its volume. We can think of it as a prism with a triangular base and a height of 8 cm, where the area of the triangle is half of a 6 cm by 10 cm rectangle. In each case we can get an expression equivalent to this for the volume (in cm³): ½×6×8×10.
TUESDAY: Here we consider how pyramids can be formed from a cuboid. The three pyramids are different, but do they have the same volume?
How obvious is it to students that the three pyramids have the same volume and so this volume is ⅓ of the volume of the cuboid - and hence can be expressed as ⅓ × base area × height? One way to access this is to consider the situation when the cuboid is a cube. Then, clearly, the three pyramids will be identical. If we now stretch the cube to make a cuboid, all the volumes will be changed by the same factor, so the pyramids, though no longer identical, will still have identical volumes.
It might reassure some students, and it can be illuminating, to assign values to the dimensions of the cuboid, as in the diagram below. They can then verify that the volume formula gives the same numerical result for each pyramid.
At this point it might be interesting to go back to Monday's wedge, which can be partitioned into two pyramids, one of which is the same as the first of the three pyramids, above. The other can be thought of as having a 6 cm by 10 cm triangular base and a height of 8 cm. The wedge and these two pyramids have volumes that are ½, ⅓ and ⅙ of the volume of the 6 cm by 8 cm by 10 cm cuboid.
WEDNESDAY: Here we compare a pyramid to one that is similar but whose dimensions (such as its edges) are only half as long. We can see that the new volume is a lot smaller, but how readily do students see that the volume is only ⅛ of the original pyramid?
In the case of a cube, it is fairly easy to see that if we halve its sides we can fit 8 such cubes into the original - but we can't do that with pyramids....
On the other hand, if students are comfortable with the volume formula for a pyramid, V₁ = ⅓abc, then if we halve each dimension we get V₂ = ⅓×½a×½b×½c = ⅛×⅓abc = ⅛×V₁.
Note: We are dealing here with the property that the volume of an enlarged object is proportional to the cube of the linear scale factor. In the same way, the area is proportional to the square of the linear scale factor, which is an issue that we look at in Week 17.
THURSDAY: This is a purely qualitative task - we are only interested in whether there is any change in the surface area of the pyramid's various faces. Of course, given that the pyramid is enveloped by a cube, we could, with the aid of Pythagoras' Rule, find expressions for the area of each face.
The triangular faces of the pyramid change shape as P moves towards Q, but does this affect their areas? If we think of the horizontal edges AB, BC, CD and DA as the bases of the triangles, then what happens to the triangles' heights? For triangles ABP and CDP the heights don't change. However, the height of ADP increases while that of BCP decreases. Does this balance out?
In the elevation view, below, the blue line segments show the heights before P has moved; the red line segments show the heights when P has moved towards Q. It can be proved (How?) that the total length of the blue lines is less than the red lines. So the total area of the pyramid's faces increases.
The volume of the slabs (in m³) is 1 + 4 + 9 + 16 + 25 + 36 + 49 = 140.
The volume of a 7 m by 7 m by 7 m pyramid is ⅓×7³ = 343÷3 = 114⅓, which is rather low because this is cutting off quite large parts of the slabs. A pyramid with dimensions of 7½ m gives a better fit (a side elevation would like this, below). Its volume (in m³) is ⅓×7½×7½×7½ = ⅓×3375÷8 = 140⅝.