Week 20

 

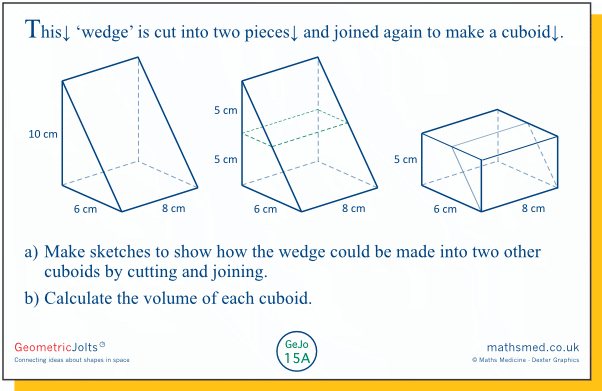
Describe translations as 2D transformations.
Apply addition and subtraction of vectors, multiplication of vectors by scalar, and diagrammatic and column representations of vectors; {use vectors to construct geometric arguments and proofs}.

Here we have collected together the final two Key Stage 4 geometry statements of 'knowledge, skills and understanding', which we address in this final week's set of tasks.

Vectors are powerful in that they allow us to apply abstract algebraic processes to geometry and thereby also expand geometric ideas. However, it is difficult to convey their power at this level of mathematics. Indeed, at this level they can often seem cumbersome and obscure when used to prove statements that already seem obvious or are amenable to more familiar and tangible geometric processes. [But see Wednesday's task, where the use of vectors provides quite an efficient way of comparing magnitudes.]

MONDAY: This task might seem rather abstract at first, but a careful examination of the diagram should help ground the task.

The task can be solved formally by constructing these simultaneous equations:
9m + 2n = 19 and m + 5n = 26.

However, we can also solve the task by inspection. Given that m and n are whole numbers, the value of n must be 1. For example, a value of 2 would give us an x-component of at least 2×9+2 which takes us too far to the right. In turn, the value of n must be 5, to give us an x-component of 19 = 1×9 + 5×2. 

TUESDAY: The aim here is to spot a relation and to express it in general form. Beyond that, students might feel it has little purpose!

Students should find the first part to be quite straightforward. If they reflect upon it, they might discern that the vector for point B has an x-component that is 18 – 10 more than that for A and a y-component that is 77 – 50 less. So the vector that maps B onto Q can be written as this:

WEDNESDAY: This task provides quite a nice mixture of the visualising activities that one associates with Euclidean geometry and the numerical/analytic approach associated with vectors.

There are simple relationships, which we can discern from the geometry of the regular octagon, between the x- and y-components of the vectors AC, CE and HF, whilst AE is the sum of AC and CE. The desired vectors are these (written as rows for typographical reasons!):
CE = (-1   1+√2),  AE = (√2   2+√2),  HF = (1   1+√2).

We already know, from the symmetry of the regular octagon, that AE is parallel to HF. The vectors confirm this, since √2×HF = (√2×1   √2×1+√2×√2) = (√2   2+√2) = AE. This also shows that AE = √2×HF. We could, of course, have determined this from what we know about the relation between the lengths of the side and diagonal of a square (see the diagram below). But interestingly here, deriving this relation using vectors is more efficient than doing so by calculating the actual lengths of AE and HF (using Pythagoras' Rule and our knowledge about the dimensions of the regular octagon).

THURSDAY: This task involves another regular polygon - the dodecagon.

The size of the exterior angles of a regular dodecagon is 30˚. This means that the magnitude of vector b is twice the magnitude of its y-component, so the magnitude is 2√3 units. [Alternatively, we could use Pythagoras' Rule: (magnitude)² = 3² + (√3)² = 9 + 3 = 12, so magnitude = √12 = 2√3.] In turn this means vector a (written as a row) = (2√3   0).  

We can use the symmetry of the regular dodecagon to relate c to b and thus determine that c = (√3  3).

Vector PS is the sum of vectors a, b and c,
so PS = (2√3+3+√3   0+√3+3) = (3+3√3   3+√3) = [1+√3]×(3   √3) = [1+√3]×b.
And so PS is parallel to b.

FRIDAY: One aim of this task is to illustrate the potential power of a vector-based approach for solving geometric problems and how this may require little more than handle-turning with relatively little thought - and which, in turn, may provide very little insight when compared to a more visual approach!

Students might need some help with understanding what is meant by 'equating the coefficients' and with appreciating the power of doing so. We have tried to make the task more accessible by providing students with the two expressions for the vector AP. However, constructing such expressions is perhaps the most interesting aspect of a vector approach, so you might want to give some students the opportunity to derive the expressions themselves.

The two expressions for AP capture the fact that:
• AP lies on AC, a vector that we know
• we can get from A to P via the point E whose position we know.

Once we equate the coefficients in the two expressions, the values of m and n emerge automatically from the solution process:
We can see that mu = nu, and so m = n.
And we can see that mv = ¼v –¼nv, so m = n = ¼ –¼n, and so ⁵⁄₄ n = ¼, and so n = ⅕ = m.

However, this algebraic approach will carry very little meaning for many of us. Compare it to the geometric approach below, which shows that m = ⅕ (below, left) and n = ⅕ (below, right).

Week 19

Know the exact values of sin 𝛉 and cos 𝛉 for 𝛉 = 30˚, 45˚, 60˚ and 90˚; know the exact value of tan 𝛉 for 𝛉 = 0˚, 45˚, 60˚.
{Know and apply the sine rule and the cosine rule to find unknown lengths and angles.}
{Know and apply Area = ½ab sinC to calculate the area, sides or angles of any triangle.} 

Here we have collected together three statements of 'knowledge, skills and understanding' and are addressing them in just one week's set of tasks. This is not ideal, but we're running out of weeks!

So we have a very quick look at some familiar trigonometric ratios and at the sine, cosine and Area-of-a-triangle rules. The tasks can generally be solved without formal trigonometry, by for example using Pythagoras' Rule and ideas about similarity. An aim of the tasks is to remind students of the origins of the formal rules, which can be done in various ways. For example, you might explicitly ask students to solve a given task in two ways - by using and by not using one of the rules. Or you might leave it open, to see what methods students spontaneously adopt, which can then be shared.

MONDAY: This task doesn't require the explicit use of trigonometric ratios, but it rests on the fact that in a right-angled triangle, if the other angles are 45˚, the triangle is isosceles, and if the other angles are 30˚ and 60˚, the length of the hypotenuse is twice the length of the shortest side. In turn, this means that the sides are in the ratio 1 : 1 : √2 and 1 : √3 : 2, using Pythagoras' Rule.

We can find the lengths of sides in the first two triangles in a step by step way (below, left) or in a more curtailed way by making direct use of the above ratios (below, right).

We could now keep repeating either of these processes. Or we could curtail things by making use of the fact that the hypotenuse of length 20√6 mm that we have just found is ½√6 times the length of the first triangle's hypotenuse of 40 mm. So if we apply this multiplier 3 more times, we get to the final hypotenuse and the value of x:
x = 20√6 × ½√6 × ½√6 × ½√6 = 20 × 36 ÷ 8 = 90.

TUESDAY: The sine rule applies to triangles. It has a very simple proof based on writing equivalent expressions for a triangle's height. We can do the same kind of thing for this special quadrilateral.

 WEDNESDAY: We can express all the key lengths in the given figure using just two variables (once we have used our knowledge of 60˚ right-angled triangles). The resulting expressions for the yellow and green areas can then be equated and simplified using Pythagoras' Rule, until the desired result falls out.

The result also falls out very neatly using the cosine rule. Consider the diagram below, where we have added two red diagonal lines that serve as hypotenuses. The total green area is equal to a² (Pythagoras' Theorem). Using the cosine rule, we can write
a² = b² + c² – 2bc cos120 = b² + c² + 2bc×½ = b² + c²  +  bc
= area of yellow squares + yellow rectangle.

As alluded to earlier, we can use first principles and write the area of the green squares as
(b + ½c)² + (½c√3)² = b² + bc + ¼c² + ¾c² = b² + c² + bc = yellow area.

WEDNESDAY Extra: Here is a variant of the above task where the the hypotenuses are already present. The task might be useful for students who are stuck on the previous task or to consolidate the earlier ideas.

We can express the area of the yellow square using Pythagoras' Theorem and the green square using the cosine rule. Or we can use first principles to find expressions for the sides of the squares (namely 6√2 cm and 6√3 cm). It turns out that the difference between the areas of the green and yellow squares is 36 cm². Put another way, the area of the green square is equal to the area of the two yellow squares, below:

 

THURSDAY: In this task it is fairly easy to see how the values of a and b change as C moves along the green arc. However, it is less easy to be certain about their product.

As point C approaches B the product ab approaches 0 since b approaches the fixed value c and a approaches 0. But what happens when C is say one-third and one-half of the way along the green arc?

One way to achieve certainty is to apply the area rule to triangle ABC:
Area of triangle ABC = ½ab sinC.

The angle C doesn't change when C moves on the green arc, so the maximum value of ab is achieved when the triangle's area is a maximum. If we take c as the base (which also doesn't change), this occurs when C is exactly halfway between A and B since the height of the triangle is then a maximum.

 

FRIDAY: Here we have two triangles with the same size base. Their third vertices are the endpoints of  radii of the same circle. How do the angles that these radii make with the base affect the triangles' heights?

The heights of the two triangles can be expressed as OPsin25˚ and OQsin50˚, or Rsin25˚ and Rsin50˚, where OP = OQ = R. The sine function is not linear, as we saw in Task 18A: it turns out that the value of sin50˚ is slightly less than 2sin25˚, and so the area of the larger triangle is slightly less than twice that of the smaller triangle.

We can say all this in condensed form using the area formula:
Area of AOP = ½rRsin25˚, area of BOP =½rRsin50˚ (where r is the radius of the smaller circle);
 2×½rRsin25˚ > ½rRsin50˚ because 2sin25˚ > sin50˚.

Week 18

Apply Pythagoras' theorem and trigonometric ratios to find angles and lengths in right-angled triangles {and, where possible, general triangles} in two {and three} dimensional figures. 

Here we have a quick look at some key trigonometric concepts.

MONDAY: It is easy to fall into the trap of thinking that trigonometric functions, in this case the sine function, are linear. If that were the case, then the suggestion in this task, that sin 70˚ = 0.966, would be true. In fact sin 70˚ = 0.940 (to 3 decimal places).

It is worth pointing out that for 'smallish' angles, the sine function is quite close to being linear. Consider this set of values. If one focusses on the first two decimal places, it is only when we get to 36˚ that it becomes clear that the values are not linear.

One way to counter Tricia's claim is to use an ad absurdum argument: If the pattern were linear, then sine 80˚ would equal 1.066 which is greater than 1.

A more grounded argument involves adding vertical lines like those below to the given diagram. If we think of the circle as having a radius of 1 unit, then the vertical lines' lengths give us the sine of the angles. As we can see, as the angles steadily increase (towards 90˚), the lines increase in ever smaller steps.

TUESDAY: This task exploits the idea that we can compare angles if we embed them in triangles that have something in common, for example if the triangles are both right-angled.

An informal, qualitative, way to solve this task is to imagine rotating the plane containing the red lines about the right-hand vertical edge of the cuboid (see the diagram below, left). As the horizontal red line overlaps a horizontal edge of the cuboid, the slanting red line intersects a horizontal edge of the top face between its two endpoints rather than at an endpoint. So the red slanting line is steeper than the green slanting line which means the red angle is larger than the green angle.

A more analytic way is to form the right-angled triangles shown below, right. The triangles have the same height, but the red triangle has a smaller base (the red and green bases are in the ratio 1 : √2). So the red angle is larger than the green angle.

You might want to make the trigonometric ideas more explicit by using this variant:

 

TUESDAY: Back to basics....

A grounded way of solving this is to consider the right-angled triangle where the length of the hypotenuse is 1 unit and the side opposite our angle is 0.6600 units. Then the length of the adjacent side, a units, is given by a² = 1 – 0.6600², so a = 0.7513 and so the tangent is 0.6600÷0.7513 = 0.879 (correct to 3 dp).

An alternative would be to use the relations sin²A + cos²A = 1 and tanA = sinA/cosA, though students are unlikely to know these. It is also heavy handed.

THURSDAY: This is quite a complex task, though its underlying aim is relatively simple. This is to convey the idea that, at this level of schooling, trigonometry is essentially a form of scale drawing (albeit one that is restricted to right-angled triangles) where someone has already done the drawing and recorded the measurements for us.

The task involves several steps. One way to break it down is like this: enlarge the original figure by a scale factor 0.2 to give the figure below-left. Then rotate, enlarge and re-attach the smaller (brown) triangle, as in the figure below-right, so that its side that had become 20 units long becomes 25.66 units long - an enlargement of scale factor 1.283. So the side that had become 23.84 units long becomes 30.59 units long.

FRIDAY: Another trigonometry-as-scale-drawing task....

This again involves several steps. One way to break it down is shown in the figures below. These involve an enlargement of scale factor 0.6 and then an enlargement (of just the rotated brown triangle) of scale factor 76.98 ÷ 71.52 = 1.076.

Week 17

Apply the concepts of congruence and similarity, including the relationships between lengths, {area and volumes} in similar figures (2) 

This is the second of two weekly sets of tasks looking at (congruence and) similarity. The approach is quantitative this time in that we use our knowledge about similar shapes to calculate lengths or areas. [A task that involves the relation between linear scale factor and volume scale factor can be found in Week 15: Task 15C]

MONDAY: Here we use the fact that the ratio of the areas of similar shapes is the square of the linear scale factor. The challenge is to find the necessary linear scale factors.

The area of triangle A (and B) is 1 cm². If we think of the base of triangle A as the side of length 1 cm, then the corresponding bases of triangles C and D are 2 cm and √(1² + 2²) = √5 cm long. So their areas are 2² times and √5² times the area of triangle A, which gives areas of 4 cm² and 5 cm².

We can confirm that 4 copies of triangle A will exactly fill triangle C, with a diagram like the one below. It is also clear that more than 4 copies are needed to fill triangle D, though it is not immediately obvious that we need exactly 5!

TUESDAY: This task is reminiscent of Task 16C. The key is to realise that P is the point such that angle ACP is equal to angle ABC. The task allows students to confirm, and perhaps become more familiar with, the relation (linear scale factor)² = area scale factor. However, it is possible that students get too immersed in the numerical detail.

Point P has coordinates (6, 1). We can confirm that angles ACP and ABC are equal by, for example, considering their tangents or, in a more grounded way, by considering the slopes of CP and BC (see the diagram, below): we can get from C to P in steps of 3-vertical, 2-horizontal and from B to C in steps of 3-horizontal, 2-vertical.

The areas of the triangles are ½×9×6 = 27 unit squares and ½×6×4 = 12 unit squares.
Their ratio is 27 : 12 = 9 : 4 = 3² : 2².

BC = √(9² + 6²) = √117 = 3√13 units and CP = √(6² + 4²) = 2√13 units.

BC : CP = 3 : 2 (we can of course  confirm this by finding the ratio of any other pair of the triangles' corresponding sides, giving the ratios 9 : 6 or 6 : 4.)

If we square the ratio (3 : 2) of corresponding sides, we get 3² : 2², the ratio of the areas.

WEDNESDAY: Here we find the linear scale factor from the area scale factor.

The principles behind this task are simple enough but students might find it difficult to coordinate the relevant information. Thus it might help to separate out and re-orientate the similar triangles ABC and CBP, as in the diagram below.

The area of ABC is 9 times the area of CBP so its sides are √9 = 3 times as long. So AB = 3×6 = 18 cm.

We can find the length of BP in two ways. Using within triangle ratios, we can state that BP : CB = BC : AB = 6 : 18 = 1 : 3, and so BP = 2 cm. Or we can make use of areas. If we refer back to the original diagram, and think of AB and BP as the bases of our similar triangles, then they have the same height, h cm. In turn we can state that ½×18×h = 45, so h = 5, and so ½×BP×5 = 5 and so BP = 2 cm.

THURSDAY: In this task we are given the area of a trapezium and asked to find the area of another. It is tempting to think these trapezia are similar, since corresponding angles are equal. However, on closer examination it is pretty clear that they are not! Are there shapes that we can use that are similar?

The key to this task is that there are three similar triangles in the diagram, two of which contain the trapezia.
The scale factor that maps triangle ABC onto ADE is (8+2)/8 = 1¼.
So the area scale factor is (1¼)² = ²⁵⁄₁₆.
And so we can write ²⁵⁄₁₆ × Area ABC = Area ABC + 9 cm²,
so ⁹⁄₁₆ × Area ABC = 9 cm²,
and so Area ABC = 16 cm².
We can now show that Area AFG = (14/10)² × 25 cm² = 49 cm²,
and so Area DFGE = 49 – 25 cm² = 24 cm².

FRIDAY: This is a somewhat quirky task. Its intrigue (for students who are fairly fluent in manipulating algebraic symbols) lies in the fact that some of the desired formulae turn out to be quite simple.

a) Face U has dimensions a and b. The corresponding dimensions for the similar face V are b and c. So we can write a : b = b : c (note that this works whether we think of the ratios as within the shapes or between them). In turn we can write c = b²/a and Area of W = ab²/a = b².

b) If the area of face U is 20 unit squares, we can write a = 20/b.
So we can write this for the area of face V: bc = bb²/a =bb²b/20 = b⁴/20.

The cuboid looks like this (using an isometric projection) for selected values of b:

 

Week 16

Apply the concepts of congruence and similarity, including the relationships between lengths, {area and volumes} in similar figures (1) 

This is the first of two weekly sets of tasks looking at congruence and similarity. The approach here is qualitative rather than quantitative in that the focus is on whether or not figures are congruent or similar, rather than on using such knowledge to calculate lengths, areas or volumes.

MONDAY: This task is quite challenging because one of the edges is curved in each shape.

 

If we flipped the smaller shapes so they overlapped their larger companion as in the diagram below, then if the pairs of shapes are similar the larger shape would be an enlargement of its companion. Is that the case? Are the curved edges 'parallel'? In a sense they are as they are arcs of concentric circles, but in the case of the yellow shapes it is not clear how points on the two arcs 'correspond'. Moreover, if we consider the ratio of corresponding straight edges, then it is clear that XA/XA' is not equal to XB/XB'. We can also argue that the corresponding 'angles' in the yellow 'triangles' are not equal. For example, the tangent to the smaller circle at A makes a different angle with XA' than the tangent to the larger circle at A'.

The diagram below shows various enlargements of the yellow 'triangle' XAB. None are similar to XA'B'.

MONDAY EXTRA: Here is an extra task that could be used to explore the idea of 'parallel arcs' further.

 

TUESDAY: This is quite a complex task, made more challenging by the fact that we don't know enough about the lengths of the shapes' sides to be able to say with certainty that a pair of shapes are congruent or similar. On the other hand, the marked angles allow us to say with certainty that some pairs of shapes are not congruent or similar.

A useful starting point is to look for corresponding angles. For example, shapes A and B have the same angle (marked o) opposite the right angle, as do shapes C, D and E.

The only two shapes that look as though they might well be congruent are C and D.

Shapes A and B look as though they might be similar, and E might well be similar to the congruent pair C and D.

WEDNESDAY: A necessary condition for two shapes to be similar is that corresponding angles are equal. For triangles this is a sufficient condition; moreover, if two pairs of corresponding angles are equal, then all three will be equal.

It is perhaps easier to find pairs of similar triangles by matching angles than by actually visualising the triangles. For example, triangles ADE and EDC are similar because they both contain one of the marked angles and they share the angle at D. If one wanted to map one of the triangles onto the other, this would involve several transformations: a reflection and a rotation (and an enlargement if one wanted to make them the same size).

Triangles ACE and ECB are also similar. Some students might choose the pair of triangles ECB and ECD as well, but this would only be true if the angles at C were right angles.

THURSDAY: This is a very subtle task and quite complex....

A powerful way of solving this task is to use the notion of enlargement. As the pairs of L-shapes have the same orientation (corresponding sides are parallel), one shape can be mapped onto the other by an enlargement if the shapes are similar. Moreover, the centre of enlargement will have to be at the top right vertex of the rectangle that envelops the two Ls (since the top edges of the two Ls lie on the top edge of the rectangle and their right-hand edges lie on the right-hand edge of the rectangle).

In the first diagram, both shapes look like 'proper' Ls, in that for each L the horizontal and vertical 'limbs' are the same thickness. However, the Ls are not similar because the limbs of the green L are the same thickness as those of the yellow L, and so would become thinker if we enlarged it to achieve the same height, say, as the yellow L. Note also that if we joined corresponding inner corners of the Ls, the line (shown in red, below) does not go through the top-right corner of the enveloping rectangle. 

For the second pair of Ls, the equivalent red line does go through the top-right corner, but the Ls are still not similar. The scale factor required to map the inner edges of the green L onto the yellow L does not map its outer edges onto the outer edges of the yellow L (see the shape with the green outline, below).

It looks as though the third pairs of L-shapes might well be similar: the red line (below) goes through the top-right corner of the enveloping rectangle and the limbs of the green L are thinner than the corresponding limbs of the yellow L.

The last pair of L-shapes are not similar, even though the limbs of the green L are thinner than the corresponding limbs of the yellow L. If we enlarge the green L so its limbs are the same thickness as those of the yellow L, the shapes don't fully overlap (below).

FRIDAY: This task again involves L-shapes but the shapes are much simpler and easier to compare. In Thursday's task, a necessary (but not sufficient) condition for two L-shapes to be similar was that for each individual L, the shape formed by the inner two edges had to be similar to the shape formed by the corresponding two outer edges. Here we have reduced the task to just comparing such pairs of rectilinear shapes.

So, for example, the first pair of L-shapes below are formed from the two inner and the corresponding two outer edges of the first yellow L-shape in Thursday's task.

The task can help students achieve greater clarity if they struggled with aspects of Thursday's task.

It turns out that all the pairs consist of similar L-shapes, except the first, where a line through the corner of each L-shape (shown in red, below) does not go through the top-right corner of the enveloping rectangle.

The last two pairs are easy to assess, as the L-shapes together with the slanting line form triangles with equal corresponding angles. If one thinks in terms of enlargement, the centre is where the line through the  corners meets the slanting line.

Week 15

Calculate surface areas and volumes of spheres, pyramids, cones and compound solids (2) 

This is the second of two weekly sets of tasks looking at surface area and volume of various kinds of solids. Here we focus on the pyramid, though we start with a 'wedge', and the tasks are mostly quantitative.

MONDAY: Here we transform a wedge-shape into a cuboid in various ways.

In the given example, the wedge has been transformed into a cuboid with the same base but half the height of the original wedge. We can also transform it into a cuboid with half the base of the original wedge but the same height, as in the examples below.  Of course, we don't need to transform the wedge to find its volume. We can think of it as a prism with a triangular base and a height of 8 cm, where the area of the triangle is half of a 6 cm by 10 cm rectangle. In each case we can get an expression equivalent to this for the volume (in cm³): ½×6×8×10.

TUESDAY: Here we consider how pyramids can be formed from a cuboid. The three pyramids are different, but do they have the same volume?

How obvious is it to students that the three pyramids have the same volume and so this volume is ⅓ of the volume of the cuboid - and hence can be expressed as ⅓ × base area × height? One way to access this is to consider the situation when the cuboid is a cube. Then, clearly, the three pyramids will be identical. If we now stretch the cube to make a cuboid, all the volumes will be changed by the same factor, so the pyramids, though no longer identical, will still have identical volumes.

It might reassure some students, and it can be illuminating, to assign values to the dimensions of the cuboid, as in the diagram below. They can then verify that the volume formula gives the same numerical result for each pyramid.

 

At this point it might be interesting to go back to Monday's wedge, which can be partitioned into two pyramids, one of which is the same as the first of the three pyramids, above. The other can be thought of as having a 6 cm by 10 cm triangular base and a height of 8 cm. The wedge and these two pyramids have volumes that are ½, ⅓ and ⅙ of the volume of the 6 cm by 8 cm by 10 cm cuboid.

WEDNESDAY: Here we compare a pyramid to one that is similar but whose dimensions (such as its edges) are only half as long. We can see that the new volume is a lot smaller, but how readily do students see that the volume is only ⅛ of the original pyramid?

In the case of a cube, it is fairly easy to see that if we halve its sides we can fit 8 such cubes into the original - but we can't do that with pyramids.... 

On the other hand, if students are comfortable with the volume formula for a pyramid, V₁ = ⅓abc, then if we halve each dimension we get V₂ = ⅓×½a×½b×½c = ⅛×⅓abc = ⅛×V₁.

Note: We are dealing here with the property that the volume of an enlarged object is proportional to the cube of the linear scale factor. In the same way, the area is proportional to the square of the linear scale factor, which is an issue that we look at in Week 17.  

THURSDAY: This is a purely qualitative task - we are only interested in whether there is any change in the surface area of the pyramid's various faces. Of course, given that the pyramid is enveloped by a cube, we could, with the aid of Pythagoras' Rule, find expressions for the area of each face.

 

The triangular faces of the pyramid change shape as P moves towards Q, but does this affect their areas? If we think of the horizontal edges AB, BC, CD and DA as the bases of the triangles, then what happens to the triangles' heights? For triangles ABP and CDP the heights don't change. However, the height of ADP increases while that of BCP decreases. Does this balance out? 

In the elevation view, below, the blue line segments show the heights before P has moved; the red line segments show the heights when P has moved towards Q. It can be proved (How?) that the total length of the blue lines is less than the red lines. So the total area of the pyramid's faces increases.

FRIDAY:A pre-calculus approach to volume? Cutting a shape into slices....

The volume of the slabs (in m³) is 1 + 4 + 9 + 16 + 25 + 36 + 49 = 140.

The volume of a 7 m by 7 m by 7 m pyramid is ⅓×7³ = 343÷3 = 114⅓, which is rather low because this is cutting off quite large parts of the slabs. A pyramid with dimensions of 7½ m gives a better fit (a side elevation would like this, below). Its volume (in m³) is ⅓×7½×7½×7½ = ⅓×3375÷8 = 140⅝.